When assessing an existing span you are able to measure dimensions such as attachment heights, and often you want to derive the stringing tension of the span, or the sag under alternative temperature conditions.
Data required for these calculations are:
- distances – span length, attachment heights (see figure 1)
- conductor type/properties
- air and conductor temperatures
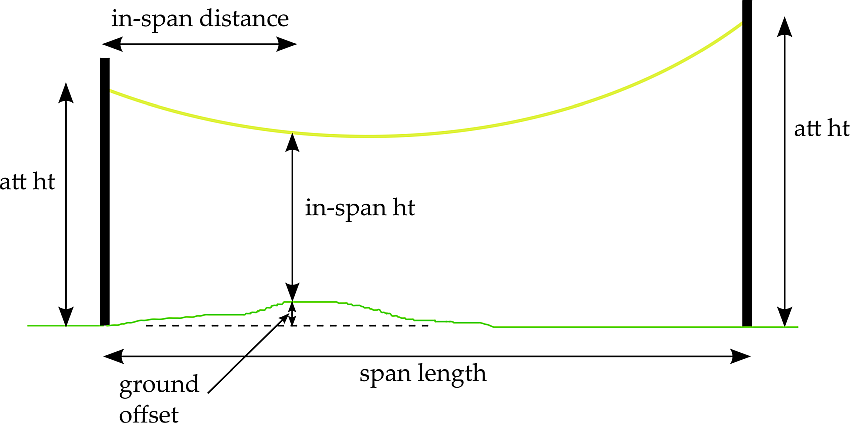
Mid-span sag can be calculated from item 1 data, by simple trigonometry. Since tension is inversely proportional to sag, the actual tension at the time of measurement can be calculated1 using the conductor properties.
Deriving stringing tension from actual tension requires accurate (or reasonably accurate) assessment of conductor temperature at the time the field data was taken, and also the Ruling Span (RS, also known as Mean Equivalent Span) for the strain section the span is part of.
Ruling span is not too difficult to calculate and is an important factor. Figure 2 shows calculation of actual tension in a 120m span of Hydrogen with a stringing tension of 7% CBL2 for a range of conductor temperatures (Standard temperature is 15°). If RS of 120m is used when the actual RS is 80m the tension is too high, leading to over-engineering of the pole design and under-estimation of sag. If RS of 160m is used the calculated tension is too low. This points out the need for accurate RS values in the design process.
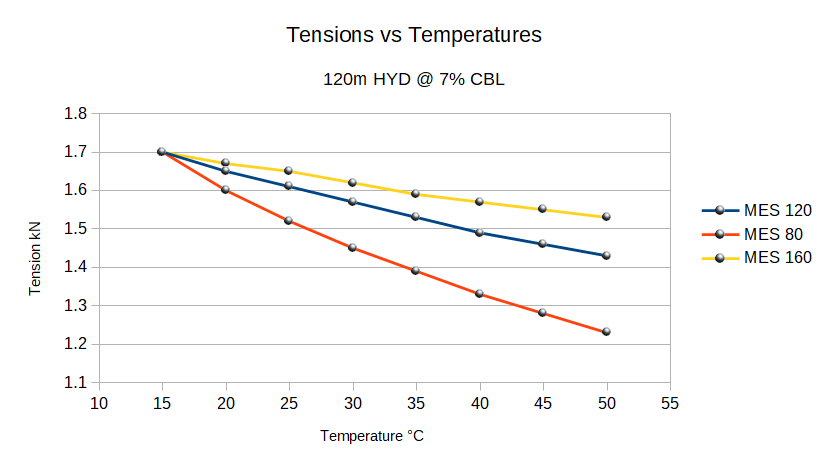
Because the procedure for “field measurements to tension” operates in reverse to the example in the previous paragraph, the outcomes are reversed. Therefore, using a RS that is higher than the actual RS results in a calculated tension that is less than actual.
Since Ruling Span is usually easy to calculate it is recommended this is done to reduce inaccuracies.
Similarly the value used for actual conductor temperature at the time of field work affects calculated tensions, also shown in Figure 2. Using an estimate for temperature that is too high leads to a stringing tension that is also higher than actual. It is difficult to arrive at an accurate conductor temperature but a higher value will be more conservative if your intention is to estimate the tipload on the pole due to the existing circuits.
The actual conductor temperature will always be higher than ambient (air) temperature, although if there is more than a light breeze most heating in the conductor due to sun or current will be counteracted by the cooling effect of the breeze.
